ORACLE

The qhychwa word "Watuq" refers to the character who reads or tells the fortune, something similar to what the term "Oracle" would represent according to the West.

The Quadratures

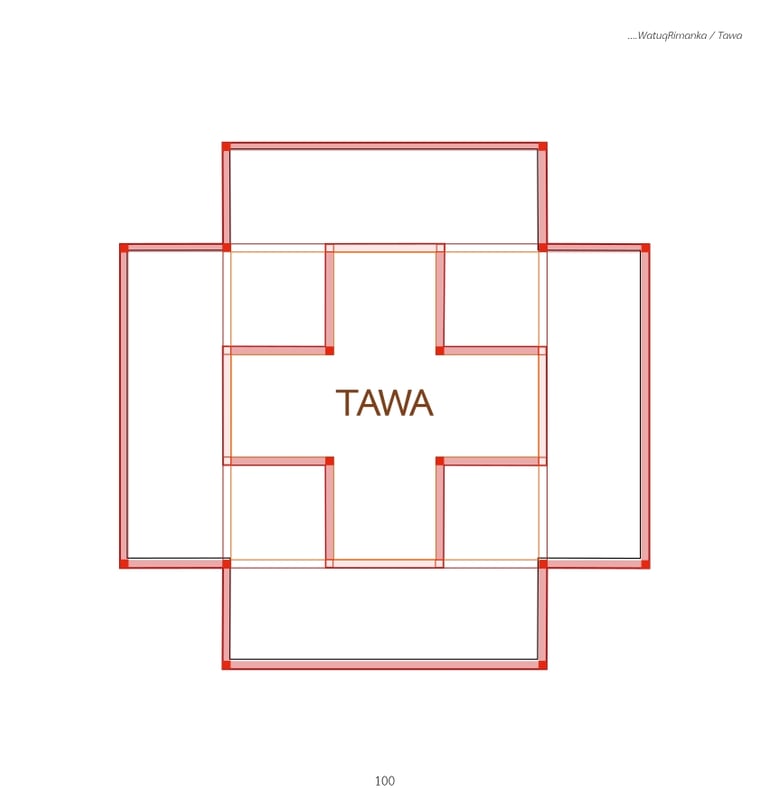
The processes known as the "Quadrature of the Circumference" and in particular, the process of the "Quadrature of the Circle", refer to the "mathematical" operations that allow us to match the perimeter and area of a circle with the perimeter and area of a quadrilateral and the square. This gives rise to the expression associated with the "Quadrature of a Circle", an expression commonly used to describe something that is very difficult or impossible to solve.

These operations progressively lead us to begin to identify and calculate the initial value of "Pi". The Greek mathematician Euclid was the "first" to demonstrate that the ratio of the circumference of a circle to its diameter was a constant value called ∏. Although there are several definitions of ∏, the most common is that ∏ is the ratio between the circumference of a circle to its diameter.

In this Willakuy or Tale we begin to find a difference or gap between the initial value of ∏ used by ancient cultures and the value "deliberately" imposed by the civilization of modern humans.

As we will show, the ancient cultures used the value of 3.1622 or √10 as an initial value and the civilization of modern humans claims that the value of "Pi" refers to a static constant value of 3.1415, with many and many decimals. This last value would only be valid if the aim was to impose a specific "geometry" on all other "geometries" and on all other forms of "life" surrounding us.

What is the Quadrature of a Circle?

The expression "Quadrature of a Circle" is a concept that has been used throughout history in different contexts, both in mathematics and in language, and in everyday "life". The "Squaring of a Circle" refers to the attempt to construct a square having the same area as a given circle, using only a ruler and a compass, that is, without using more advanced measuring instruments, such as a grading ruler or calculating instruments.

The expression "Squaring the Circle" had its origin in ancient Greece, where mathematicians were faced with the "impossibility" of performing this task using only a plain ruler and a compass. Despite this, the search for a solution to this problem became an intellectual challenge that has persisted throughout the centuries.

The "Quadrature of the Circle", within a "mathematical" context, is considered an unsolvable problem, because – according to the principles of "classical geometry" – it is not possible to construct a square with the same exact area as that of a circle using only a ruler and a compass.

The expression "Quadrature of a Circle" is used to refer to the difficulty or impossibility of solving a problem. It is used as a metaphor to refer to a task that is extremely difficult or impossible to perform. Despite being an "insoluble" mathematical problem, the "Quadrature of the Circle" has been a source of inspiration for research and progress in various areas. For example, it has driven the development of numerical methods and approach techniques that allow us to obtain increasingly more precise values of the area of a circle.